Bernoulli’s Theorem from Work-Energy Theorem:

Let us consider a tube AB of an incompressible fluid of density \( \rho \). Let \( \alpha_1 \), \( \alpha_2 \) and \( v_1 \), \( v_2 \)and \( P_1 \), \( P_2 \) are respectively the cross sectional area, velocities and pressure at the ends \( A \) and \( B \).

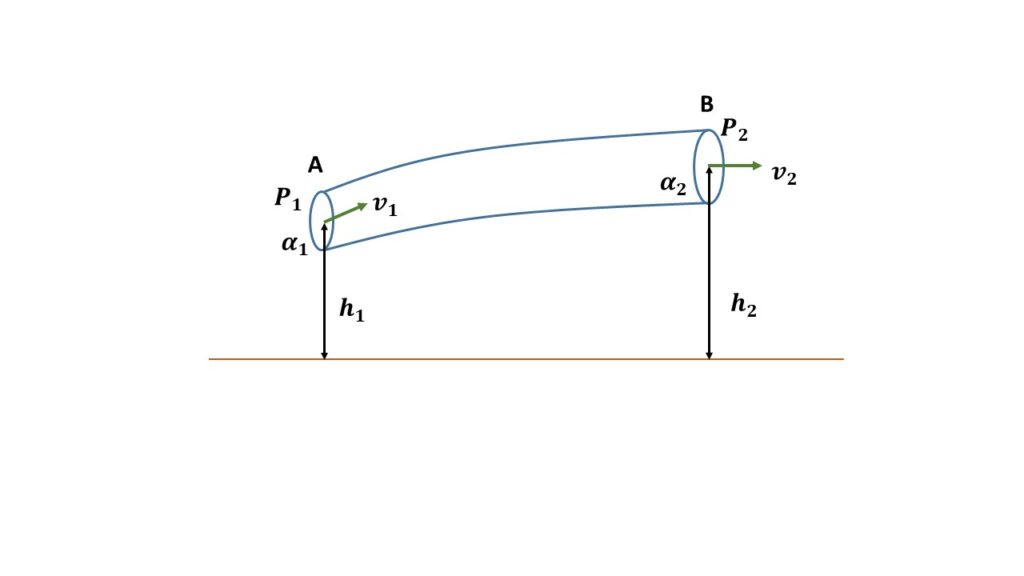
So the volume of fluid entering A per second is \( \alpha_1v_1 \), and the volume of fluid leaving B per second is \( \alpha_2v_2 \)

The work done per second on the mass of the fluid entering A is \( P_1\alpha_1v_1 \) and the work done by the fluid at B per second is \( P_2\alpha_2v_2 \).

So the work done by the fluid in raising against the gravity from \( h_1 \) to \( h_2 \) is \( mg(h_2-h_1) \). Now net work done on the fluid per second is

\( W=P_1\alpha_1v_1-P_2\alpha_2v_2-mg(h_2-h_1)\\=P_1V_1-P_2V_2-mg(h_2-h_1) \)

where, \( V_1 \) and \( V_2 \) are the volume of the fluid flowing through \( A \) and \( B \) per second.

Since the fluid is incompressible, so \( V_1=V_2=V=\frac{m}{\rho} \)

Thus \( W=(P_1-P_2)\frac{m}{\rho}-mg(h_2-h_1) \)

Now the change in the kinetic energy of the fluid is \( \delta{E_k}=\frac{1}{2}m{v_2}^2-\frac{1}{2}m{v_1}^2 \)

From work-energy theorem, the total work done on a system is equal to increas in its kinetic energy, \( W=\delta{E_k} \)

or, \( (P_1-P_2)\frac{m}{\rho}-mg(h_2-h_1) = \frac{1}{2}m{v_2}^2-\frac{1}{2}m{v_1}^2 \),

or, \( (P_1-P_2)\frac{1}{\rho}-g(h_2-h_1) = \frac{1}{2}{v_2}^2-\frac{1}{2}{v_1}^2 \),

or, \( P_1+\frac{1}{2}\rho{v_1}^2+\rho{g}h_1=P_2+\frac{1}{2}\rho{v_2}^2+\rho{g}h_2 \)

or, \( P+\frac{1}{2}\rho{v^2}+h\rho{g}=constant \)

or, \( \frac{P}{\rho}+\frac{1}{2}v^2+hg=constant \)

This is Bernoulli’s theorem.

Thus the sum of the gravitational head, pressure head and velocity head is constant through the motion during steady flow of an incompressible viscous fluid.