Orbital velocity of an artificial satellite:

Let us consider an artificial satellite revolves around the earth with a velocity \( v \), at a height \( h \) from the surface of the earth. Mass of the earth is \( M \) and radius is \( R \).

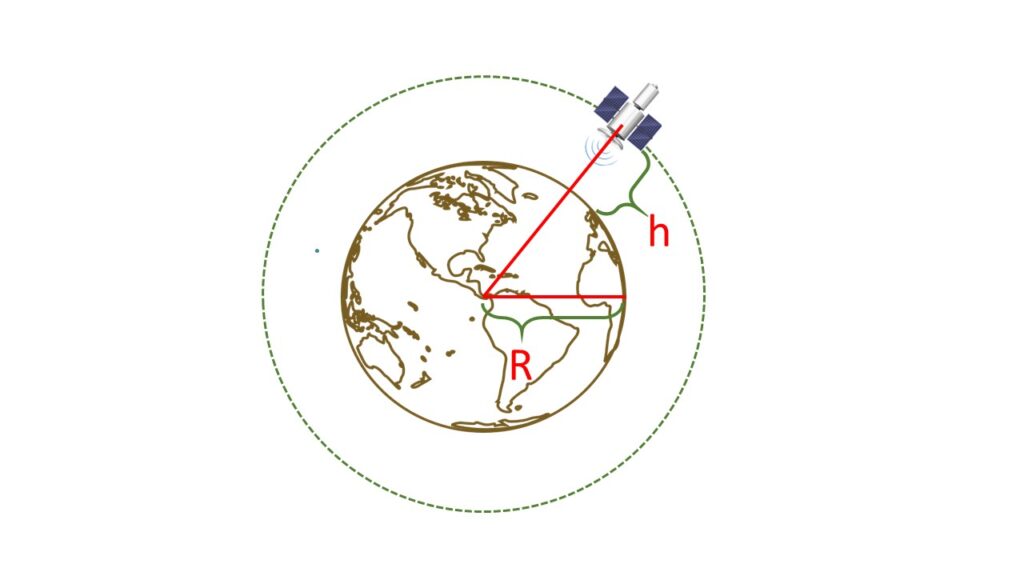
Here, the gravitational force of attraction is equal to centrifugal force on the satellite.

\( \frac{GMm}{{(R+h)}^2}=\frac{mv^2}{(R+h)} \\or,\ v=\sqrt{\frac{GM}{R+h}} \)

where \( G \) is the gravitational constant.

If the satellite revolves close around the earth, then we can say that \( R+h\simeq{R} \).

Therefore \( v=\sqrt{\frac{GM}{R}}=\sqrt{\frac{gR^2}{R}} \)

or, \( v=\sqrt{gR} \), where \( g=\frac{GM}{R^2} \) is the acceleration due to gravity.