Equivalent simple pendulum:

We know that the time period of oscillation of a compound pendulum is

\( \displaystyle{T=2\pi\sqrt{\frac{K^2+l^2}{gl}}}\tag{1} \)
[ To know the derivation of this equation (CLICK HERE) ]

where, \( g \) is the acceleration due to gravity, \( l \) is the distance between the centre of gravity \( G \) and point \( O \), \( K \) is the radius of gyration.

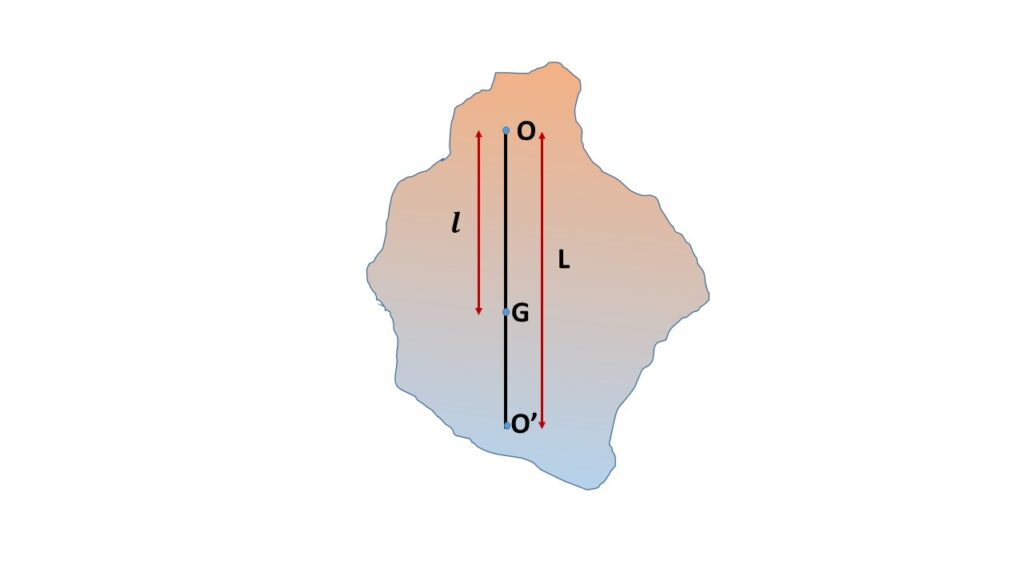
If \( L \) be the length of the simple pendulum then the time period of oscillation of the simple pendulum is given by

\( \displaystyle{T=2\pi\sqrt{\frac{L}{g}}}\tag{2} \)

From equations (1) & (2) we get

\( \displaystyle{L=\frac{K^2+l^2}{l}} \)

or, \( \displaystyle{L=\frac{MK^2+Ml^2}{Ml}} \)

or, \( \displaystyle{L=\frac{I}{Ml}} \)

Where, \( I \) is the moment of inertia of the compound pendulum about an axis through the point O.

So \( L \) which is represented by OO’, is the length of the simple pendulum, equivalent to the compound pendulum.

O is called centre of suspension and O’ is called the centre of oscillation.